Primary Electrical Formulas

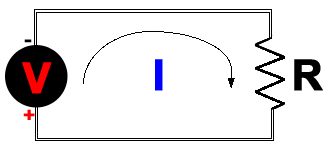
The formula below shows the basic realtionship of the main factors in an electrical circuit. It is known as Ohm's Law. Ohm's Law in more exact definition deals with the relationship between voltage and current in an ideal conductor. This relationship states that: The potential difference (voltage) across an ideal conductor is proportional to the current through it.

I = E / R
Explanation of Terms

E - stands for voltage, the unit is the Volt.
I - stands for current, the unit is the Amp.
R - stands for resistance/impedance, the unit is the Ohm.

Joule's Law (below) is derived (using variable substitution) from Ohm's basic formula using a new measurement that includes the value for power. It should be apparent why the term volt-amp means watt.

P = I * E
Explanation of Newest Term

P - stands for power, the unit is the Watt.

Refer to these formulas and the diagram below when desiging your system to see how these equations come into play. In the diagram below, V is used for voltage instead of E.

Simple Circuit


From all of the above, consider the following:
P = I (squared) * R



Simplified explanation of Power (wattage), Current (amperage), and Force (voltage)


A balistics analogy may assist to explain the physics involved above. For a given shotgun load, there are a number of equally sized pellets in the load propelled from the barrel at a determined velocity (voltage equivalent). A higher pellet velocity is similiar to a higher voltage. Voltage describes the force of each electron as it travels through a wire. The number of pellets in the load is similiar to the current (amperage). A shotgun load with more pellets is like higher amperage. More pellets exiting the barrel (at the same velocity) is similar to a higher number of electrons traveling through a wire cable per second. The total power of the shotgun load is the total of the force (velocity) of each pellet multiplied by the number of pellets (current equivalent). This total power is similar to the wattage in an electrical circuit. A greater wattage simply means more power. Greater wattage could be due to greater velocity from each pellet, or a greater number of pellets, or both.




Click to recommend
website to others